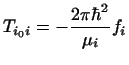

Next: 5 Distorted Wave Born
Up: Methods of Direct Reaction
Previous: 3 Model Schrödinger Equation
Greens function methods may also be used to solve the coupled equations, and furthermore
suggest various approximations which simplify the solution methods in many special cases
of interest. First, therefore, we present in this section several exact results using
T-matrix integrals derived from Greens function analysis. In the following section
5 we examine various consequent approximations that are often still accurate.
If the full wave function  were known, then the scattering amplitude fi for
the
were known, then the scattering amplitude fi for
the
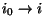 reaction may be found from the T-matrix by the equivalence
reaction may be found from the T-matrix by the equivalence
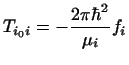 |
|
|
(20) |
so that, written in terms of the transition amplitudes, the expression for the cross
section becomes
 |
|
|
(21) |
Expressions for the T transition amplitudes may be derived by
using either plane waves or distorted waves in the exit channel.
In addition, for transfer reactions where the channel Hamiltonians are different
in the initial and final channels, we have a further choice of
using either post or prior forms of the coupling.
The post form uses the form of Hi for the exit channel, and the prior form
the Hi0 from the entrance channel.
The plane-wave post matrix element is
| Ti0i |
= |
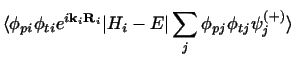 |
(22) |
| |
= |
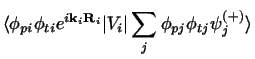 |
(23) |
where the (+) superscript in 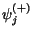 indicates that they are found
with plane incoming waves in the i0 channel.
A prior matrix element uses the Hamiltonian Hi0 of the initial channel. Direct substitution
in Eq. (22), however, affords no simplifications, so we insert it in the matrix
element for the time-reversed reaction, and derive
indicates that they are found
with plane incoming waves in the i0 channel.
A prior matrix element uses the Hamiltonian Hi0 of the initial channel. Direct substitution
in Eq. (22), however, affords no simplifications, so we insert it in the matrix
element for the time-reversed reaction, and derive
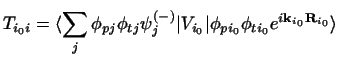 |
|
|
(24) |
where the (-) superscript in 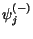 indicates that
it has an incident plane wave along
indicates that
it has an incident plane wave along 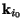 and incoming spherical
waves e-ikiRi in all channels.
Distorted-wave expressions may be found by replacing the exponential factors on
the left sides by one-channel scattering waves (
and incoming spherical
waves e-ikiRi in all channels.
Distorted-wave expressions may be found by replacing the exponential factors on
the left sides by one-channel scattering waves (
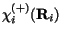 on the right sides
and
on the right sides
and
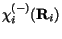 on the left), found with some distorting potential Wi by
on the left), found with some distorting potential Wi by
![$ [T_i + W_i - E_i]\chi_i({\bf R}_i)=0$](img87.gif) .
The distorted-wave post matrix element is then
.
The distorted-wave post matrix element is then
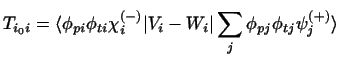 |
|
|
(25) |
and the equivalent prior form is
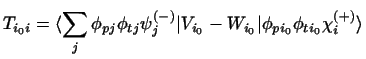 |
|
|
(26) |
The distorting potential Wi may be real or complex without affecting the validity
of these matrix elements.
All these four expressions are so far identical, and exactly equivalent to solving
the coupled equations directly and using Eq. (20).


Next: 5 Distorted Wave Born
Up: Methods of Direct Reaction
Previous: 3 Model Schrödinger Equation
Prof Ian Thompson
2006-02-08